Slater orbital
In quantum chemistry, Slater-type orbitals (STOs) are functions used as atomic orbitals. They are named after the physicist John C. Slater, who introduced them in 1930[1]. STOs are primarily used in the computation of wave functions of atomic and diatomic systems. In ab initio methods applied to molecules with more than two centers, certain integrals arise (three- and four-center two-electron integrals) that cannot be integrated analytically within an STO framework. Larger molecules can be handled by means of STOs, however, in certain semi-empirical methods, where the more difficult integrals are neglected.
Contents |
Radial part
STOs have the following radial part:
where
- n is a natural number that plays the role of principal quantum number, n = 1,2,...,
- N is a normalization constant,
- r is the distance of the electron from the atomic nucleus, and
- ζ is a shielding constant related to the effective charge of the nucleus, the nuclear charge being partly shielded by electrons.
The normalization constant is computed from the integral
Hence
With this factor the radial part is normalized to unity. That is, explicitly we have,
Angular part
It is common to use the real form of spherical harmonics as the angular part of the Slater orbital. A list of Cartesian real spherical harmonics is given in this article. In the article on hydrogen-like orbitals is explained that the angular parts can be designated by letters: s, p, d, etc. The first few Slater type orbitals are listed in the next subsection. We use s for l = 0, p for l = 1 and d for l = 2. Functions between square brackets in the list are normalized real spherical harmonics. These functions are given in Cartesian form but, when expressed in spherical polar coordinates, are functions of the colatitude angle θ and longitudinal (azimuthal) angle φ only (r is divided out). If we denote such a function by Y(θ, φ), then the following normalization condition is satisfied
Explicit form
A list of explicit STOs up to and including 3d orbitals follows.
Reference
- ↑ J.C. Slater, Atomic Shielding Constants, Phys. Rev. vol. 36, p. 57 (1930)
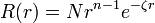

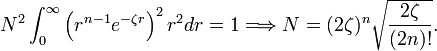
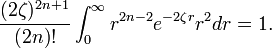
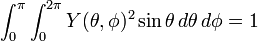
![\begin{align}
1s &= 2\zeta^{3/2} e^{-\zeta r}\Big[\sqrt{\frac{1}{4\pi}}\Big]
= \sqrt{\frac{\zeta^3}{\pi}} e^{-\zeta r} \\
2s &= \frac{2}{3}\sqrt{3} \zeta^{5/2}\, r \,e^{-\zeta r}\Big[\sqrt{\frac{1}{4\pi}}\Big]
= \sqrt{\frac{\zeta^5}{3\pi}}r \,e^{-\zeta r} \\
2p_x &= \frac{2}{3}\sqrt{3} \zeta^{5/2}\, r \,e^{-\zeta r} \Big[\sqrt{\frac{3}{4\pi}} \frac{x}{r} \Big] = \sqrt{\frac{\zeta^{5}}{\pi}}\, x \,e^{-\zeta r} \\
2p_y &= \frac{2}{3}\sqrt{3} \zeta^{5/2}\, r \,e^{-\zeta r} \Big[\sqrt{\frac{3}{4\pi}} \frac{y}{r} \Big] = \sqrt{\frac{\zeta^{5}}{\pi}}\, y \,e^{-\zeta r} \\
2p_z &= \frac{2}{3}\sqrt{3} \zeta^{5/2}\, r \,e^{-\zeta r} \Big[\sqrt{\frac{3}{4\pi}} \frac{z}{r} \Big] = \sqrt{\frac{\zeta^{5}}{\pi}}\, z \,e^{-\zeta r} \\
3s &= \frac{2}{15}\sqrt{10} \zeta^{7/2}\, r^2\,e^{-\zeta r}\Big[\sqrt{\frac{1}{4\pi}}\Big]
= \sqrt{\frac{2\zeta^7}{45\pi}} r^2\,e^{-\zeta r}\\
3p_x &= \frac{2}{15}\sqrt{10} \zeta^{7/2}\, r^2\,e^{-\zeta r}\Big[\sqrt{\frac{3}{4\pi}} \frac{x}{r} \Big] = \sqrt{\frac{2\zeta^7}{15 \pi}} \,r\, x\, e^{-\zeta r} \\
3p_y &= \frac{2}{15}\sqrt{10} \zeta^{7/2}\, r^2\,e^{-\zeta r}\Big[\sqrt{\frac{3}{4\pi}} \frac{y}{r} \Big] = \sqrt{\frac{2\zeta^7}{15 \pi}} \,r\, y\, e^{-\zeta r} \\
3p_z &= \frac{2}{15}\sqrt{10} \zeta^{7/2}\, r^2\,e^{-\zeta r}\Big[\sqrt{\frac{3}{4\pi}} \frac{z}{r} \Big] = \sqrt{\frac{2\zeta^7}{15 \pi}} \,r\, z\, e^{-\zeta r} \\
3d_{z^2} &= \frac{2}{15}\sqrt{10} \zeta^{7/2}\, r^2\,e^{-\zeta r} \Big[\sqrt{\frac{5}{4\pi}} \frac{3z^2-r^2}{2r^2}\Big] = \frac{1}{3}\sqrt{\frac{\zeta^7}{2\pi}}\,(3z^2-r^2)\,e^{-\zeta r} \\
3d_{xz} &= \frac{2}{15}\sqrt{10} \zeta^{7/2}\, r^2\,e^{-\zeta r} \Big[\sqrt{\frac{5}{4\pi}}\sqrt{3}\frac{xz}{r^2}\Big] = \sqrt{\frac{2\zeta^7}{3\pi}} \,xz\,e^{-\zeta r} \\
3d_{yz} &= \frac{2}{15}\sqrt{10} \zeta^{7/2}\, r^2\,e^{-\zeta r} \Big[\sqrt{\frac{5}{4\pi}}\sqrt{3}\frac{yz}{r^2}\Big] = \sqrt{\frac{2\zeta^7}{3\pi}} \,yz\,e^{-\zeta r} \\
3d_{x^2-y^2} &= \frac{2}{15}\sqrt{10} \zeta^{7/2}\, r^2\,e^{-\zeta r} \Big[\sqrt{\frac{5}{4\pi}}\frac{1}{2}\sqrt{3}\frac{x^2-y^2}{r^2}\Big] = \sqrt{\frac{\zeta^7}{6\pi}} \,(x^2-y^2)\,e^{-\zeta r} \\
3d_{xy} &= \frac{2}{15}\sqrt{10} \zeta^{7/2}\, r^2\,e^{-\zeta r} \Big[\sqrt{\frac{5}{4\pi}}\sqrt{3}\frac{xy}{r^2}\Big] = \sqrt{\frac{2\zeta^7}{3\pi}} \,xy\,e^{-\zeta r} \\
\end{align}](images/math/c/2/a/c2ab5d859606d08173bbd2b911fc1dca.png)