Talk:Number

[edit] Defining numbers

"The concept of number is one of the most elementary, or fundamental notions of mathematics. Such elementary concepts cannot be defined in terms of other concepts" – Not at all. In the mainstream 20 century mathematics, the fundamental notions are "set" and "belong"; others, including numbers, are defined. Pedagogy is another matter... --Boris Tsirelson 14:22, 23 November 2011 (EST)

I confess the sentence to which you object was written by myself (along with the first two paragraphs, though not the rest of the article). I was aware of the Frege-Russell definition of number as well as Wittgenstein's critique thereof (he charged that the Frege-Russell definition was circular). When I edited the CZ article, I wrote to one of the math folks and asked him to examine it as I was not quite sure of myself. He evidently saw nothing wrong and left it as I wrote it.

Anyway, any definition must employ concepts and terms other than the one being defined. So the question is: which concepts are to be considered fundamental and which derivative? If the concept of number as derived from set concepts is useful in the sense that non-trivial information is thereby arrived at, then perhaps set and member are more fundamental (provided the definition is not circular as Wittgenstein held).

Incidentally, the approach I employed seems to be in line with that of Soviet mathematicians, at least as I am aware of them, though perhaps I was looking at material which was more pedagogical than theoretical.

Whatever is done with this, I hope the bibliographical and on-line references can direct readers to more information on the subject.

JFPerry 21:51, 23 November 2011 (EST)

I see. A fair article should mention different approaches, I think so. Unfortunately I am acquainted with only one approach - that of the mainstream 20 century mathematics; and yes, set theory is considered quite successful (which does not mean that everyone is satisfied with it). Philosophers may have different attitudes, of course. Pedagogy also. And some, non-mainstream, mathematicians. (About Soviet math: I was myself a Soviet mathematician till the age 40, but I did not note the trend that you mention. I guess it is not specific to Soviet, but to something else, probably pedagogy as you also guess.) --Boris Tsirelson 01:26, 24 November 2011 (EST)

Technically, there is no notion "number" at all in mathematics. 'Today, it is no longer easy to decide what counts as a "number." The objects from the original sequence of "integer, rational, real, and complex" are certainly numbers, but so are the p-adics. The quaternions are rarely referred to as "numbers," on the other hand, though they can be used to coordinatize certain mathematical notions. ...' (Gouvêa, the last paragraph.)

Thus, the "Number" article should be a lightweight, nontechnical survey, with links to more detailed and technical articles "Natural number", "Integer", "Rational number", "Real number", "Complex number" etc. But anyway, potentially misleading and controversial formulations are undesirable. --Boris Tsirelson 04:11, 24 November 2011 (EST)

Some relevant links to WP: wp:Natural number#Formal definitions, wp:Integer#Construction, wp:Rational number#Formal construction, wp:Real_numbers#Definition. wp:Complex number#Formal construction. --Boris Tsirelson 06:38, 24 November 2011 (EST)

(unindent) The article on Number, by V.I. Nechaev in the Encyclopedia of Mathematics (http://eom.springer.de/N/n067900.htm) begins by defining number to be "A fundamental concept in mathematics, which has taken shape in the course of a long historical development." However, later in the same article, it is stated: "Throughout the 19th century, and into the early 20th century, deep changes were taking place in mathematics. Conceptions about the objects and the aims of mathematics were changing. The axiomatic method of constructing mathematics on set-theoretic foundations was gradually taking shape." I'm not sure what to make of that. It doesn't seem entirely consistent.

Here are a couple of references to the Frege-Russell definition of number as critiqued by Wittgenstein.

• Boudewijn de Bruin, "Wittgenstein's Objections Against the Frege-Russell Definition of Number" (http://www.philos.rug.nl/~debruin/wittgenstein_number.html)

• Boudewijn de Bruin, "Wittgenstein on Circularity in the Frege-Russell Definition of Cardinal Number? (http://philmat.oxfordjournals.org/content/16/3/354.full.pdf?keytype=ref&ijkey=ml44Z5Czycjruli)

JFPerry 18:35, 24 November 2011 (EST)

Nice. Thus, we have (a) the non-set-theoretic mathematics, which was the only mathematics before 20 century, and is still widely used in teaching (to non-mathematicians — as a rule; to mathematicians — often on the undergraduate level; however, I have only a slight idea of various teaching systems), and (b) the set-theoretic mathematics (dominant nowadays in the mainstream mathematics research). Natural numbers are fundamental in the non-set-theoretic mathematics, and defined in the set-theoretic mathematics. Other numbers need not be treated as fundamental already before the 20 century, according to the famous phrase of Kronecker (1823 – 1891) "God made integers; all else is the work of man" (see wp:Leopold Kronecker). However, for now I do not know, to which extent they were still treated as fundamental. --Boris Tsirelson 02:18, 25 November 2011 (EST)

To stay closer to home, see Leopold Kronecker for the quotation.--Paul Wormer 04:54, 25 November 2011 (EST)

Indeed! --Boris Tsirelson 05:13, 25 November 2011 (EST)

[edit] Writing a number

"An irrational number can not be written as a fraction, and can indeed not be written out fully at all." — Really? In which sense? "The numbers π and 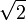are both irrational." — Are they written in this phrase? What exactly is meant here by written? It depends on the used language. In some languages 1/3 cannot be written. In some languages 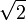can. --Boris Tsirelson 14:40, 23 November 2011 (EST) (A copy of my remark on CZ of 4 June 2010.)

Boris, what part of the quoted sentence do you disagree with? Assuming that we agree that √2 is an irrational number, do you disagree with the first part: "√2 cannot be written as a fraction", or with the second part: "√2 cannot be written in full", or with both parts? IMHO the language implied in the sentence is the basic mathematical language as taught in high schools and colleges. It seems to me that the first part is correct (definition irrational number), and that the second part is incorrect only if you want to be finicky about it (because √2 writes the irrational number in full after all), but obviously the author means to say that a decimal expansion of √2 does not terminate. Or, maybe do I misunderstand your objection completely? --Paul Wormer 11:29, 24 November 2011 (EST)

You do understand... The first part is correct, indeed.

The second part is quite unclear, however. "The basic mathematical language as taught in high schools and colleges" includes the radical, and so, the very "√2" is just written, in full, in this language.

"obviously the author means to say that a decimal expansion of √2 does not terminate" – are you sure? Did you take my hint about 1/3? The decimal expansion of 1/3 does not terminate! The author should say clearly what he/she really mean.

Finicky, yes. Being a mathematician, I am finicky all the time (and intend to continue this way). Of course I guess what the author mean; but his/her way to say it makes me unhappy. (Or should we also leave spelling errors uncorrected when it is easy to guess what is meant?) --Boris Tsirelson 11:54, 24 November 2011 (EST)

Now, a venomous question: what about the number 0.110100100010000100000100000010...? Can it be written out fully, or not? Is it substantially worse than, say, 0.10101010...=10/99, or not so much? --Boris Tsirelson 12:52, 24 November 2011 (EST)