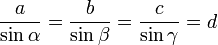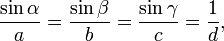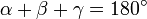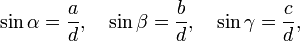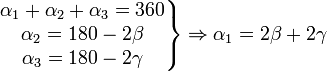Law of sines

In trigonometry, the law of sines (also known as sine rule) relates in a triangle the sines of the three angles and the lengths of their opposite sides,

or

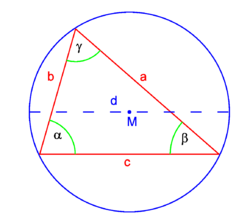
where d is the diameter of the circumcircle, the circle circumscribing the triangle. The angles and the lengths of the sides are defined in Fig. 1 for an acute-angled triangle.

From the law of sines follows that the ratio of the sines of the angles of a triangle is equal to the ratio of the lengths of the opposite sides.

The rule is useful to determine unknown angles and sides of a triangle in any of the following three cases:

• One side, the opposite angle, and one adjacent angle are given.
• One side and two adjacent angles are given.
• Two sides and an angle not included by the sides are given.

The rule

may have to be applied in such a determination.

The use of the law of sines is complementary to the use of the law of cosines.

Proof of sine rule

The easiest proof, that gives ratios and simultaneously the relationship with the diameter of the circumcircle, is purely geometric, not algebraic.

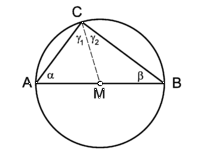
First it is recalled that any triangle, with its base coinciding with the diameter of its circumcircle, has a top angle of 90°, see Fig. 2. In this figure the angle γ₁+γ₂ = ∠ACB is equal to 90°. This follows because the lengths of the line segments AM, BM, and CM are equal (and equal to the radius of the circumcircle). Hence γ₁ = α and γ₂ = β Since γ₁ + γ₂ + α + β = 180°, it follows that the top angle ∠ACB = 90°.

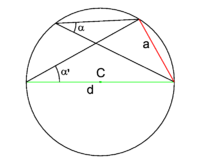
Consider next Fig. 3. The angle α with vertex on the circle is arbitrary. Given α and the chord a, the angle α′ is drawn as in Fig. 3. It has as lower arm the diameter of the circle so that the upper arm of α′, which ends at the intersection of the chord a and the circle, is perpendicular to the chord a by the result just discussed.

Note that for angle α′ it holds that: 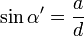   (Fig. 3), where d is the diameter of the circumcircle and a is the length of the chord opposite α′. The two angles, α and α' have the chord a in common and have their vertices on the circumference of the same circle. A well-known theorem (see below) of plane geometry states that in that case α = α' , so that it follows that the angle α has the same sine as α'.

Likewise it follows for the angles in Fig. 1:

where d is the diameter of the circumscribed circle. This proves the sine rule.

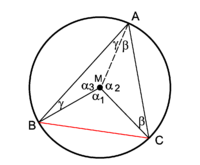
Remains to be shown that two angles that a share a chord and have a vertex on the same circle are equal. Consider to that end Fig. 4. The angle ∠BMC = 2∠BAC = 2γ+2β. Indeed, the triangles CMA and BMA are both isosceles (have two equal sides, the radius of the circle) and hence the base angles are equal. Now,

Clearly, if one moves the point A along the circle, the top angle ∠BAC of the triangle with base BC remains equal to ½α₁ (half of ∠BMC), which proves that all angles, sharing chord BC and having the vertex on the circle, are equal.

External link

Life lecture on Sine rule