Hyperelliptic curve

From Knowino

Jump to: navigation, search

In algebraic geometry and in the theory of Riemann surfaces a hyperelliptic curve is an algebraic curve $C$ of genus greater than 1, which admits a double cover 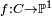$\scriptstyle f:C\to\mathbb{P}^1$ . These curves are among the simplest algebraic curves: they are all birationally equivalent to curves of the form $y 2 - f (x)$ in the affine plane, where $f (x)$ is a polynomial in $x$ , and the degree of $f (x)$ is either twice the genus of the curve plus 2, or twice the genus of the curve plus one.

If a double cover 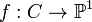$f:C\to\mathbb{P}^1$ exists, then it is the unique double cover 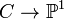$C\to\mathbb{P}^1$ , and it is called the "hyperelliptic double cover". The involution induced on the curve $C$ by interchanging between the two "sheets" of the double cover is called the "hyperelliptic involution". The divisor class of a fiber of the hyperelliptic double cover is called the "hyperelliptic class".

Weierstrass points

By the Riemann-Hurwitz formula the hyperelliptic double cover has exactly $2 g + 2$ branch points. For each branch point $p$ we have $h 0 (2 p) = 2$ . Hence these points are all Weierstrass points. Moreover, we see that for each of these points 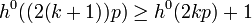$h^0((2(k+1))p)\geq h^0(2kp)+1$ , and thus the Weierstrass weight of each of these points is at least 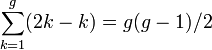$\sum_{k=1}^g (2k-k)=g(g-1)/2$ . However, by the second part of the Weierstrass gap theorem, the total weight of Weierstrass points is $g (g 2 - 1)$ , and thus the Weierstrass points of $C$ are exactly the branch points of the hyperelliptic double cover.

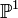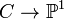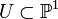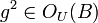Given a set $B$ of $2 g + 2$ distinct points on $\mathbb{P}^1$ , there is a unique double cover of $C\to\mathbb{P}^1$ whose branch divisor is the set $B$ . From an algebro-geometric point of view one can construct the curve $C$ by taking the $P r o j$ of the sheaf whose sections $g$ over an open subset $U\subset\mathbb{P}^1$ satisfy $g^2\in O_U(B)$ .

The plane model

File:Hyperelliptic plane.png

A plane model for a hyperelliptic curve of genus 3. Two Weierstrass points have non-real coordinates, and one is at infinity.

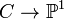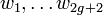If the $2 g + 2$ branch points of a hyperelliptic double cover $C\to\mathbb{P}^1$ are $w_1,\ldots w_{2g+2}$ then C is birational to the plane curve 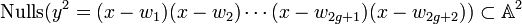$\mbox{Nulls}(y^2=(x-w_1)(x-w_2)\cdots(x-w_{2g+1})(x-w_{2g+2}))\subset\mathbb{A}^2$ , where if one of the branch points is infinity, we omit the corresponding term in the product. 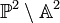The closure of this curve in the projective plane has a singularity on the line $\mathbb{P}^2\setminus\mathbb{A}^2$ .

Curves of genus 2

If the genus of $C$ is 2, then the degree of the canonical class $K C$ is 2, and $h 0 (K C) = 2$ . Hence the canonical map is a double cover. The Jacobian variety of such a curve is an Abelian surface.

The canonical system

If $p$ is a rational point on a hyperelliptic curve, then for all $k$ we have 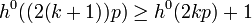$h^0((2(k+1))p)\geq h^0(2kp)+1$ . Hence we must have 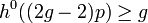$h^0((2g-2)p)\geq g$ . However, by Riemann-Roch this implies that the divisor $(2 g - 2) p$ is rationally equivalent to the canonical class $K C$ . Hence the canonical class of $C$ is $g - 1$ times the hyperelliptic class of $C$ , and the canonical image of $C$ is a rational curve of degree $g - 1$ .

Level 2 structure

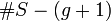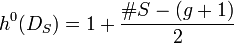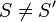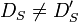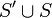If $S$ is a set of at most $g + 1$ Weierstrass points of $C$ such that $\# S-(g+1)$ is even, then $D_S:=[S]+\frac{\#S-(g+1)}{2}H_C$ is a theta characteristic of $C$ ; i.e. $2 D S \sim K C$ is in the Picard group of $C$ . Moreover, it can be shown that $h^0(D_S)=1+\frac{\#S-(g+1)}{2}$ , and if there are two such sets $S\neq S'$ , then either $D_S\neq D_S'$ or $S'\cup S$ is the set of all Weierstrass points on $C$ .

Every theta characteristics may be obtained in this way: Indeed if we count each set $S$ together with its complementary set in the set of Weierstrass points (and then divide by 2) then the combinatorial description above tells us that any partition of the set of Weierstrass points into two sets such that the difference between the cardinalities is divisible by 4, induces a theta characteristic. In this way we count a total of 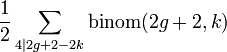$\frac{1}{2}\sum_{4|2g+2-2k}\mbox{binom}(2g+2,k)$ distinct theta characteristics . This combinatorial sum is $2 2 g$ which is the number of theta characteristics on a curve of genus $g$ . Hence our description exhausts all the theta characteristics.

File:Family bitangents.png

a family of bitangents to canonical curves of genus 3 degenerate to a line connecting images of two Weierstrass points of a canonical image of a hyperelliptic curve of genus 3

In genus 2, there are 6 Weierstrass points. Each of them is an odd theta characteristic. There are $binom(6,3) = 20$ three-tuples of distinct Weierstrass points, and hence there are $20 / 2 = 10$ odd theta characteristics, as expected. It is interesting to understand the geometrical meaning of pairs of Weierstrass points: if $p 1, p 2$ are two distinct Weierstrass points on $C$ then $p 1 + p 2 - H C$ is a 2-torsion point on the Jacobian of $C$ , in this way we can express all the $24 - 1 = 15 = binom(6,2)$ non-trivial 2-torsion points on this Jacobian. 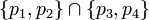Moreover, if $p 3 + p 4 - H C$ is another (and different) 2-torsion point, then the $W e i l p a i r i n g$ between the two 2-torsion points is given by $\{p_1,p_2\}\cap\{p_3,p_4\}$ .
In genus 3, there are 8 Weierstrass points. The even theta characteristics are given by the empty set and by partitions of these 8 points into two distinct sets - hence we get $1 + binom(8,4) / 2 = 36$ even theta characteristics. The odd theta characteristics are given by the $binom(8,2) = 28$ pairs of Weierstrass points. The canonical map in this case maps the curve $C$ to a double cover of a plane conic. Consider a family of canonically embedded genus 3 curves with parameter $t$ given by $Nulls(Q 2 + t F)$ , where we identify the double conic $Q 2$ with the canonical image of $C$ and where the Weierstrass points are the intersection points of 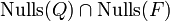$\mbox{Nulls}(Q)\cap \mbox{Nulls}(F)$ . Then it can be shown that the "limit" of the bitangents of the curves in the family (which are the odd theta characteristics) are the lines connecting pairs of images of Weierstrass points on $C$ .

Moduli of hyperelliptic curves

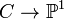Since for any set $B$ of $2 g + 2$ points on $\mathbb{P}^1$ there is a unique double cover $C\to\mathbb{P}^1$ with branch divisor $B$ , the coarse moduli space of hyperelliptic curves of genus $g$ is isomorphic to the moduli of $2 g + 2$ points on 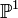$\mathbb{P}^1$ , up to projective transformations. 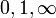However, as there are more than three points in $B$ , there is a finite non-empty subset of $\mbox{Aut}(\mathbb{P}^1)=\mbox{PGL}_2$ that sends three of the points in $B$ to $0,1,\infty$ . Thus, the moduli of space which parametrizes $2 g + 2$ distinct points on 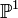$\mathbb{P}^1$ up to projective transformations, is a finite quotient of the space of distinct $2 g - 1$ points on 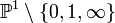$\mathbb{P}^1\setminus\{0,1,\infty\}$ . Specifically this space is an irreducible affine scheme of dimension $2 g - 1$ .

Compactifications of the moduli

There are two standard "natural" compactifications of the moduli of $n$ distinct points on 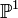$\mathbb{P}^1$ . One is the binary forms compactification 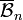$\overline{\mathcal{B}}_n$ : the GIT quotient of homogeneous polynomials of degree $n$ in two variables by the group $P G L 2$ acting on the linear span of the variables. The second compactification is the Deligne-Mumford compactification of the moduli of pointed curves 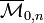$\overline{\mathcal{M}}_{0,n}$ . For the case $n = 2 g + 2$ Avritzer and Lange constructed a surjective morphism 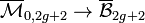$\overline{\mathcal{M}}_{0,2g+2}\to\overline{\mathcal{B}}_{2g+2}$ , thus relating these compactifications in the hyperelliptic case. 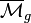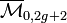Finally, the closure of the locus of hyperelliptic curves in the moduli stack $\overline{\mathcal{M}}_g$ of curves of genus $g$ , is a degree $1 / 2$ cover of $\overline{\mathcal{M}}_{0,2g+2}$ .

References

E. Arbarello, M. Cornalba, P. Griffiths, J. Harris: Geometry of Algebraic Curves, I. Grund. series, vol. 267, Springer. ISBN 0387909974 (theta characteristics on hyperelliptic curves)
D. Avritzer, H. Lange The moduli spaces of hyperelliptic curves and binary forms Math Z. Volume 242, Number 4, 2002
I. Dolgachev Invariant theory ISBN 0521525489 (binary forms compactification)
J. Harris and D. Morison Moduli of curves ISBN 0387984291 (degeneration of bitangents)

Some content on this page may previously have appeared on Citizendium.

Hyperelliptic curve

Contents

Weierstrass points

The plane model

Curves of genus 2

The canonical system

Level 2 structure

Moduli of hyperelliptic curves

Compactifications of the moduli

References

Personal tools

Namespaces

Variants

Views

Actions

Search

Navigation

Community

Toolbox